GREENWICH MERIDIAN mystery
Alright, just how impossible is that?
Notice that the latitude was practically spot on… mere feet north of the exact centre line of the pyramid. The fact that the longitude was off by about 350 yards of centre is hardly a ‘miss’. We need to understand that in 1609 the basic science of how to calculate longitude was largely unknown. Galileo was still wrestling with the problem when he died 25 years after Shakespeare’s passing.
Gerard Mercatur’s World Map of 1569 was the most accurate at the time and set the standard as the projection model used universally from then on. There’s hardly a school classroom in any country on earth that doesn’t have a Mercatur Projection World Map hanging on its wall. Nevertheless the longitude settings between Greenwich and Giza were off by 7º east on his first 1569 version. That translates to about 490 miles on the ground.
Gerard Mercatur’s World Map of 1569
y 1599 longitude calculation had improved considerably as indicated by Edward Wright’s Chart of the World on Mercatur’s Projection (sometimes known as the Wright-Molyneux Map). It was hailed both for its extraordinary accuracy and honesty. Where previous cartographers had put in all kinds of wild, squiggly speculation whenever coastline details were unknown at the time, Wright had truthfully depicted hundreds of miles with straight lines indicating, in effect… we just don’t know yet.
Relevant to our topic, Shakespeare shows he’s aware of this by referencing it in Twelfth Night having Maria joke of Malvolio: “He does smile his face into more lynes, then is in the new Mappe, with the augmentation of the Indies.” In other words he smiles but doesn’t know a thing!
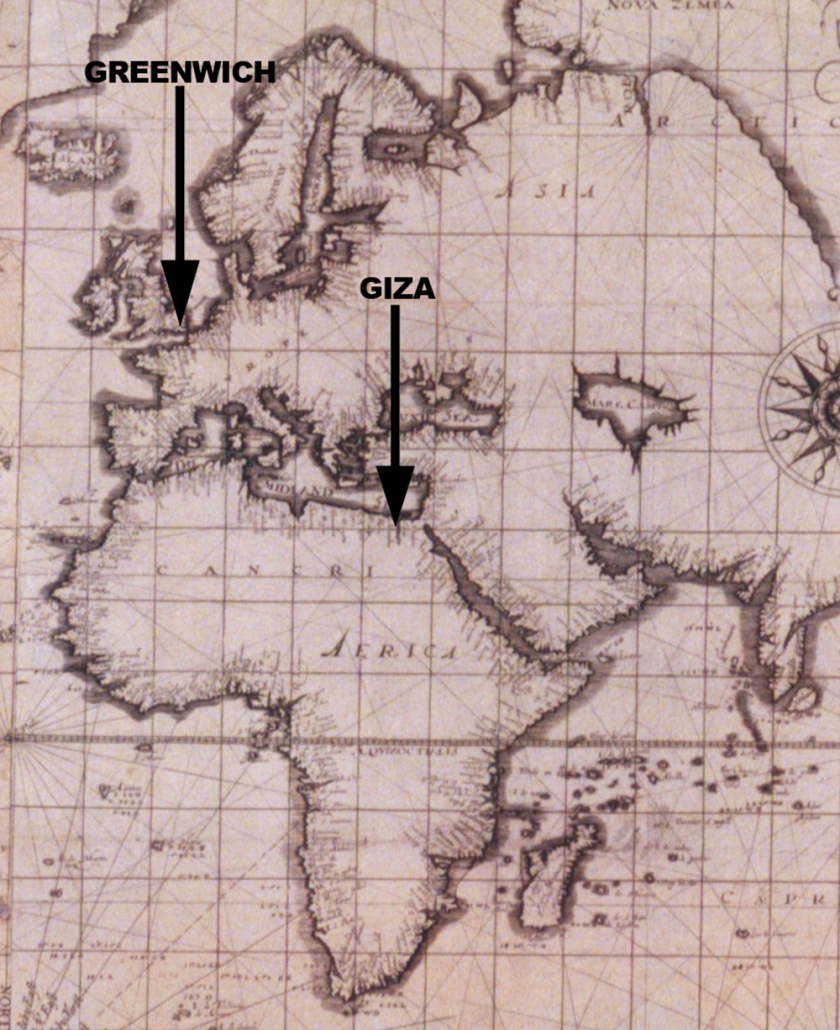
Wright-Molyneux Map of 1599
It’s interesting then that the longitude discrepancy from Greenwich to Giza had by then been reduced to about 1º. It was an enormous improvement in just 30 years but even so… you wouldn’t want to set out on camel-back in the hot desert with limited water looking for the Great Pyramid and find your GPS tracker was still 70 miles off target! The idea that someone could encrypt the longitude to be accurate to within 350 yards of its centre in 1609 is frankly astonishing.
The real point is there’s a far more esoteric level of impossibility going on here. Look closely at the longitude lines in the two maps. It isn’t clear where the 0º Prime Meridian point is located but you can see clearly that none of the vertical meridians are going directly through Greenwich. That’s because no uniform 0º position had yet been established from which to measure longitude east or west. Generally it was from a position about 25º west of Greenwich, but even that varied as the science of navigation was in its infancy and territories argued endlessly over who had the right to choose. Politics and diplomacy move at a glacial pace. Greenwich was not selected as the 0º longitude location until the International Prime Meridian Conference was held in Washington D.C…. in 1884!
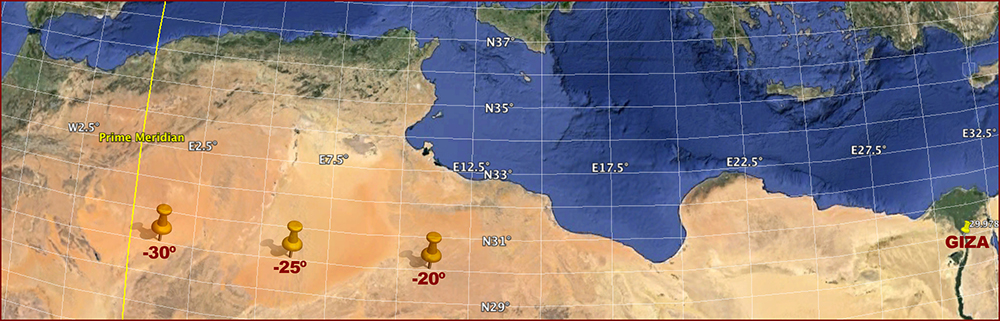
So what’s really going on? Obviously there will be those who’ll claim that because Greenwich was not the Prime Meridian location back then this discovery of 31.1299º is meaningless. It’s at this juncture that I would ask those so inclined to try their best to listen to Einstein and be a child for a couple more chapters. Of course if that’s not possible you can stay with the 0º that was most in use back then. Just FYI though, in the Google Earth map below there are pins indicating what the popular Prime Meridians of the 16th and 17th centuries would have ended up pointing us to if they were still in use today. The most common was about -25º west of Greenwich. 31.1299º east of that meridian would have led us directly to… sand! The other common variant was about -20º west of Greenwich. Sand again. It’s pretty much the same for -30º west of Greenwich too. In fact, you can plainly see that for 2,500 miles straight west of Giza there’s not a town, a river, a lake, a forest, or a Pizza Hut. It’s basically just sand all the way. Lots of it.
“Alack, sir, I am sand-blind; I know you not.”
— The Merchant Of Venice
So before you bury your head in everything west of the Great Pyramid, ask yourself one question. Would someone so brilliant… someone who knew not only the basics like π and √2 but the complex twin constants like e/e-1, ø/ ø-1, √5/√6, √3/γ, plus the twin primes constant, ß2… someone who knew how to interweave them all, to an accuracy of at least three decimal places, into a 3:4:5 triangle… someone who could cryptographically embed two angles to reveal a very special latitude and longitude… would they go to all that trouble so that for their final act they could point out a very specific pile of sand?
Or this?…

I’m just sayin’.
All we know for sure is that this phenomenon, whatever it is, is profoundly accurate, beautiful, and provocative. Someone went to incomprehensible lengths to calculate and design all this. And then hide it for “eyes not yet created” to o’er read (sonnet 81). We should probably take it very seriously. There really are more things in heaven and earth, Horatio.
Click to expand menu below.

